#VisualRatios

My sixth grade students are currently diving into ratios. Naturally, we discussed the part-whole relationship of fractions we had been looking at, and how it connected to the part-part relationship of ratios. The image below was projected onto the board, accompanied by the prompt “Make some true statements about these squares.”

Students made statements such as:

• “It’s 33.33 recent red”

• “It’s 50% [or 1/2] black”

• “1/6 are green”

Then students were challenged to make true part-part statements, such as 2 red for every 3 black. Images like this are good to start with because all of the squares are equally sized and can therefore be compared readily. The next image I showed looked like this:

Students quickly came up with the ratio of green to yellow (2:2), but were dissatisfied feeling this wasn’t descriptive enough. At this point they used the partitioning skills they’d been practicing to come up with 2:12, because

L: “There are 2 green triangles, and if we split the yellows into triangles, there will be 12.”

My class was ready for a challenge and was well-positioned to develop and extend their proportional thinking. Below are a few images they looked at in groups.

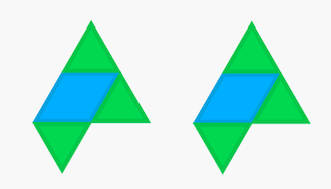
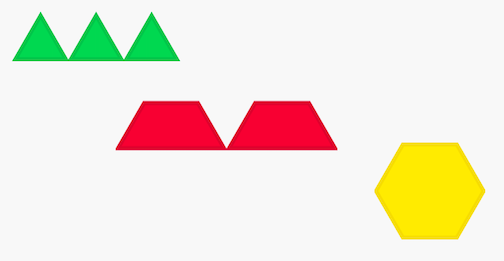
Images like these are interesting to consider because they require spatial reasoning and partitioning — the same skills that are used in a myriad of mathematical experiences. One image in particular sparked their discovery of equivalent ratios:

N: Ms. Dawson something clicked — I think [the ratio of red to yellow] is 1:2, because two red can fit in a yellow.

M: I was thinking it was 1/2:1, because one red is 1/2 of a yellow.

N: Couldn’t it also be 2:4, because there are two red and if we split the yellow into the same size pieces?

This was by far the best lesson I taught that day.

I will be posting a dropbox link with all of the #VisualRatio images I’ve made to Twitter on Tuesday, February 26.